天津东泰科技始终坚持“科技创新、精益求精、用户至上、以人为本”的经营理念,凭借良好的产品质量和市场信誉,使得公司客户遍布全国的石油、化工、煤化工行业。

产 品 简 介
01澳门网上博彩公司官网
ELC系列智能澳门网上博彩公司官网(以下简称照明控制器)产品是由澳门博彩股份公司官网创始团队在上世纪90年代在国内率先提出用于石化、化工等行业的装置照明系统、高架厂房照明系统、路灯照明系统、广场照明系统的一种目的在于延长灯具使用寿命和节电的系列产品,本照明控制器可实现对照明供电电压的软起动、稳压供电、升降压供电、过流报警、定时自动调节开关灯时间(参照天文年历进行)等功能。针对不同灯具及用户的需求,公司分别提供使用三种技术路线的三个系列的照明控制器供用户选择。其中:ELC-SM系列照明控制器的稳压技术是采用可控硅斩波技术实现的;ELC-B系列照明控制器的稳压技术是采用多抽头自耦变压器经快速多路同步开关进行无载(或称电压零点调压)调压控制技术实现的;ELC-BR系列照明控制器的调压技术是通过IGBT+补偿变压器进行精密控制升降压来实现的;ELC-BTR系列照明控制器的调压技术则是通过多抽头自耦变压器+补偿变压器进行补偿式稳压的照明控制器。这三种照明稳压控制器具有用户可设定的内部时钟,用以作为照明控制器的时间参考基准。所有的照明控制器均具有断电保护功能,内部时钟及用户设定参数不受照明控制器电源系统断电的影响。照明控制器的三相电压输出独立且可以达到100%不平衡。
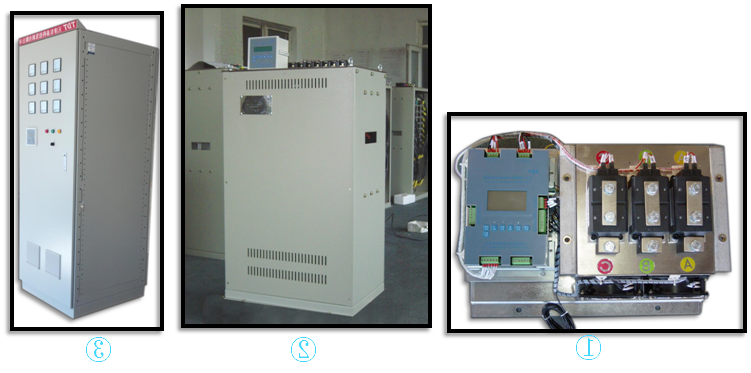 ①ELC-SM 系列照明控制器、②ELC-B 系列照明控制器/柜、③ELC-B 系列照明控制器/柜
①ELC-SM 系列照明控制器、②ELC-B 系列照明控制器/柜、③ELC-B 系列照明控制器/柜 02电动机群分批再启动控制装置
DYZQ-xxx系列电动机再启动控制装置亦是由澳门博彩股份公司官网创始团队在上世纪90年代在国内与中石油(当时的中石化)抚顺石化公司石油二厂率先联合开发出来用于石化行业抗晃电、抗低电压事件的确保连续生产不中断运行的控制系统。通过采用工业控制计算机、嵌入式控制系统、DCS及现场总线技术,确保再启动控制系统本身的运行可靠性和动作的准确性。其与目前行业内所采用的抗闪络模块以及马保的抗晃电功能相比具有无可比拟的技术先进性与安全可靠性。绝不会因为实施再启动控制而造成供电系统的崩溃。
DYZQ-xxx系列电动机抗晃电再启动控制柜的运行设置简单易行,非常便于生产现场的相关技术“零基础”人员掌握其使用。
DYZQ-xxx系列电动机抗晃电再启动控制柜采用先进的前台与后台两级控制系统结构。工业控制计算机作为控制系统的后台子系统,用于实现系统参数设定、控制过程设定、系统状态显示、历史数据记录及与上层全厂计算机监控系统的网络连接。前台DCS系统则是DYZQ-xxx系列电动机抗晃电再启动控制柜的控制核心,所用的操作系统是由我公司自行开发,拥有自主知识产权的封闭式的实时操作系统,只要前台DCS系统的电源有电(在线式UPS供电),DYZQ-xxx系列电动机抗晃电再启动控制柜就会对被晃电及低电压事件中断运行的电动机实施再启动控制。这种先进的前后台两级控制系统结构,有力的保证了DYZQ-xxx系列电动机抗晃电再启动控制柜的工作可靠性,它避免了以往其它电动机再启动控制柜生产厂家所使用的一级工业控制PC机系统结构所带来的致命缺陷(已有众多的失败教训,诸如:PC机操作系统死机;无法确保晃电信号的采集等)。
 电动机群分批再启动控制装置
电动机群分批再启动控制装置 03低压综合保护器
我公司于2004年即自主研发并成功应用于石化、煤化工行业的低压智能综合保护器。共有DYZB-100和DYZB-500两个系列的产品。DYZB-100系列低压智能综合保护器为紧凑型显示控制一体机系列。其中DYZB-101A用于低压电动机的过负荷反时限、接地、堵转、零序、缺相、不平衡、欠电压等监测保护,并适用于增安型防爆电机的Te时间保护(一种特殊的过负荷保护控制)。低压智能综合保护器还具有故障数据录波的功能。此外,还具有运行时间的累计、启动次数的累计、电动机再启动控制等功能。DYZB-102A则用于低压馈电回路的过负荷、接地、缺相、不平衡、欠电压、漏电等监测保护,并具有电能计量功能。
DYZB-500系列为分体式低压综合保护器。其有多个分型号以适应用户的多方位需求。其中DYZB-501x用于低压电动机的过负荷反时限、接地、堵转、零序、缺相、不平衡、欠电压等监测保护,并适用于增安型防爆电机的Te时间保护,其还具有故障数据录波的功能。此外,DYZB-501x低压综合保护器还具有运行时间的累计、启动次数的累计、电动机再启动控制功能等。DYZB-502x则用于低压馈电回路的过负荷、接地、漏电、缺相、不平衡、欠电压等监测保护,并具有电能计量功能。
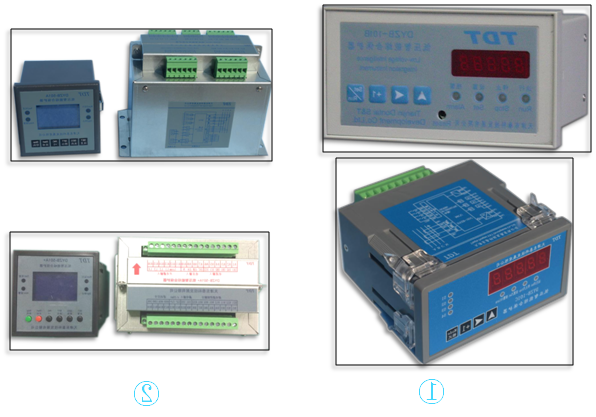 ①DYZB-100 系列低压智能综合保护器、②DYZB-500 系列低压智能综合保护器
①DYZB-100 系列低压智能综合保护器、②DYZB-500 系列低压智能综合保护器 04高性能多功能表
DGNB-B型多功能表是我公司针对电力系统、工矿企业、公共设施、智能大厦的电力监控需求而设计的计算机网络式电力仪表。它能测量所有的常用电力参数,如三相电流、电压、有功功率、无功功率、功率因数、电度等;有功数据精度可达0.5级,无功数据精度可达2级。因为该电力仪表不但具有8路4~20mA的电流换输出,还具有完善的联网通信功能,所以我们称之为网络电力仪表。它非常适合于实时电力监控系统。
 高性能多功能表
高性能多功能表05澳门网上博彩公司官网
我公司在2008年就研发生产了双冗余主机的澳门网上博彩公司官网IMCS系列(也称为澳门网上博彩公司官网),其主要功能在于负责对所在变电所相关低压用电设备的数据采集及运行状态的监测。变配电网络采用计算机监控系统的目的,就是为了保证供配电的安全性、可靠性、连续性,提高运行和自动化调度的管理水平,以便于及时发现、记录和故障分析。IMCS澳门网上博彩公司官网的基本功能包括数据采集,事件顺序记录、制表等,监控系统相关的数据采集设备主要为主二次断路器、主二次数显表、电机保护器、线路保护器、数显表、变频器、应急电源装置(EPS)、不间断电源装置(UPS)等。
 澳门网上博彩公司官网
澳门网上博彩公司官网 











